Tangent to a conic

The two intersection points of a line with a conic coincide if the discriminant of equation (2.12) is zero. This can be written as

$$S({\tt m},{\tt m}')-S({\tt m})S({\tt m}')=0 \enspace .$$

If the point 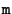${\tt m}$ is considered fixed, this forms a quadratic equation in the coordinates of 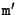${\tt m}'$ which represents the two tangents from 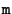${\tt m}$ to the conic. If 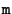${\tt m}$ belongs to the conic, $S({\tt m})=0$ and the equation of the tangents becomes

$$S({\tt m},{\tt m}')= {\tt m}^\top {\bf C} {\tt m}' = 0 \enspace ,$$

which is linear in the coefficients of 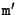${\tt m}'$. This means that there is only one tangent to the conic at a point of the conic. This tangent 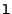${\tt l}$ is thus represented by :

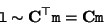$${\tt l} \sim {\bf C}^\top {\tt m} = {\bf C} {\tt m}$$ (B13)