From projective or affine to metric

In some cases it is needed to upgrade the projective or affine representation to metric. This can be done by retrieving the absolute conic or one of its associated entities. Since the conic is located in the plane at infinity, it is easier to retrieve it once this plane has been identified (i.e. the affine structure has been recovered). It is, however, possible to retrieve both entities at the same time. The absolute quadric ${\Omega}^*$ is especially suited for this purpose, since it encodes both entities at once.

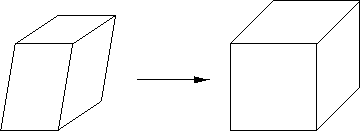
Every known angle or ratio of lengths imposes a constraint on the absolute conic. If enough constraints are at hand, the conic can uniquely be determined. In Figure 2.4 the cube of Figure 2.1 is further upgraded to metric (i.e. the cube is transformed so that obtained angles are orthogonal and the sides all have equal length).

Figure 2.4: Affine (left) and metric (right) representation of a cube. The right angles and the identical lengths in the different directions of a cube give enough information to upgrade the structure from affine to metric.

Once the absolute conic has been identified, the geometry can be upgraded from projective or affine to metric by bringing it to its canonical (metric) position. In Section 2.3.2 the procedure to go from projective to affine was explained. Therefore, we can restrict ourselves here to the upgrade from affine to metric. In this case, there must be an affine transformation which brings the absolute conic to its canonical position; or, inversely, from its canonical position to its actual position in the affine representation. Combining (2.23) and (2.20) yields

$$\Omega^* \sim \left[\begin{array}{cc} {\bf A} & {\tt a} \\ ... ...{\bf A}{\bf A}^\top & 0_3 \\ 0_3^\top & 0 \end{array} \right]$$ (B35)

Under these circumstances the absolute conic and its dual have the following form (assuming the standard parameterization of the plane at infinity, i.e. $W=0$):

$$\omega_\infty = {\bf A}^{-\top}{\bf A}^{-1} \mbox{ and } \omega_\infty^* = {\bf AA}^{\top}$$ (B36)

One possible choice for the transformation to upgrade from affine to metric is

$${\bf T}_{AM}= \left[ \begin{array}{cc} {\bf A}^{-1} & 0_3 \\ 0_3^\top & 0 \end{array} \right]$$ (B37)

where a valid ${\bf A}$ can be obtained from $\Omega ^*$ by Cholesky factorization. Combining (2.25) and (2.37) the following transformation is obtained to upgrade the geometry from projective to metric at once

$${\bf T}_{PM} = {\bf T}_{AM} {\bf T}_{PA} = \left[ \begin{ar... ...} {\bf A}^{-1} & 0_3 \\ {\tt\pi}_\infty & 1\end{array} \right]$$ (B38)